Pythagoras Theorem:
In a right triangle, the square on the hypotenuse is equal to the sum of the squares on the other two sides.
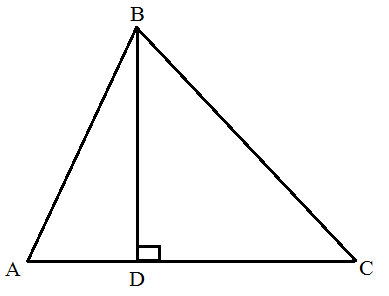
| Given: A right-angle triangle ABC in which ∠B = 90° To prove: AC2 = AB2 + BC2 Construction: From B draw BD ⊥ AC Proof: In triangles ADB and ABC, we have ∠ADB = ∠ABC [Each equal to 90°] and ∠A = ∠A [common] So, by AA-similarity, we have △ADB ~ △ABC ⇒ AD/AB = AB/AC [∵ corresponding sides of similar triangles are proportional] ⇒ AB2 = AD x AC ……….(i) In △s BDC and ABC, we have ∠CDB = ∠ABC [Each equal to 90°] and ∠C = ∠C [common] So by AA- similarity, we have △BDC ~ △ABC ⇒ DC/BC = BC/AC [∵ corresponding sides of similar triangles are proportional] ⇒ BC2 = AC x DC ……….(ii) Adding (i) and (ii), we get AB2 + BC2 = AD x AC + AC x DC ⇒ AB2 + BC2 = AC (AD + DC) ⇒ AB2 + BC2 = AC x AC ⇒ AB2 + BC2 = AC2 Hence, AC2 = AB2 + BC2 |
Converse of the Pythagoras Theorem:
In a triangle, if the square on one side is equal the sum of the squares on the remaining two, the angle opposite to first side is a right angle.
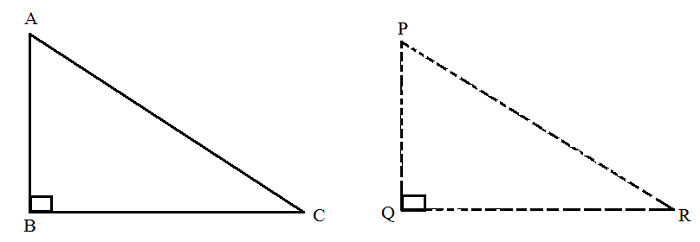
| Given: △ABC such that AC2 = AB2 + BC2 To prove: ∠ABC = 90° Construction: Construct △PQR such that PQ = AB, QR = BC and ∠PQR = 90° Proof: In △PQR, ∠PQR = 90° [By construction] ∴ PR2 = PQ2 + QR2 [By Pythagoras Theorem] or PR2 = AB2 + BC2 [∵ PQ = AB, QR = BC, by construction] But AC2 = AB2 + BC2 [given] ∴ PR2 = AC2 ⇒ PR = AC Now in △ABC and △PQR, AB = PQ [By const.] BC = QR [By const.] AC = PR [Proved above] ∴ △ABC ≅ △PQR [SSS congruency theorem] Then ∠B = ∠Q [Correspoding parts of congruent triangles] But ∠Q = 90° [By constructive]] Hence ∠B = 90° [Proved] |






Comments (No)