Logarithms and its Laws:
You know that 8 is the third power of 2 or 81 is the fourth power of 3. The relation between the base and the product is thus clear.
We consider the example 34 = 81. Here, 4 is the index of power to which the base 3 is to be raised in order to be equal to 81. 4 in this case, is called the logarithm of 81 to the base 3. The word has been derived from the Greek words Logos and Arithmos, meaning ratio and number. Logarithms was devised by the Scottish mathematician John Napier (1550 – 1617). Logarithms use the idea of base and power- and are utilized in computations with numbers.
| Thus, 23 = 8 ⇒ 3 = logarithm of 8 to the base 2. 102 = 100 ⇒ 2 = logarithm of 100 to the base 10. |
Hence, the logarithm of a number with respect to a real base (≠ 0, ± 1) is the index of the power to which the base is to be raised in order to be equal to that number.
According to the definition, the two statements ax = m and logam = x are equivalent i.e., ax = m ⇒ logam = x. Here, logam is read as “logarithm of m to the base a”.
Two systems of logarithms are in use. If the base is 10, then the logarithm is called the common logarithm. In a common logarithm, the base is not always written.
Thus, log101000 is conveniently written as log1000.
The other system of logarithms is known as the natural logarithm. In this case, the base is the sum of the infinite series i.e.
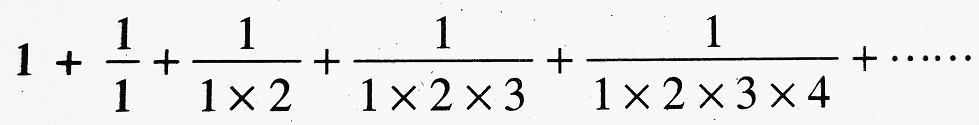
The series is denoted by e. Natural logarithms are generally denoted by ln in order to differentiate them from common logarithms which are denoted by log. Thus, ln x means logex.
Laws of Logarithms:
The sheer boredom of carrying out lengthy computations while doing multiplication and division is made simple by the use of logarithms. A few laws of logarithms have been deduced based on the laws of indices. In these laws, we shall take the logarithm to a base a (where a is a positive number, a ≠ 1).
(i) The logarithm of the product of two numbers is equal to the sum of the logarithms of the numbers to the same base i.e. loga(mn) = logam + logan (Law of Product).
Proof: Let logam = p and logan = q
∴ ap = m and aq = n
∴ mn = ap . aq = ap + q
∴ p + q = loga(mn)
∴ logam + logan = loga(mn)
The law can be generalized to the product of more than two numbers.
Thus, loga (m . n . p . q …….) = logam + logan + logap + logaq + …….
(ii) The logarithm of the quotient of two numbers is equal to the logarithm of the numerator minus the logarithm of the denominator with respect to the same base i.e. loga(m/n) = logam – logan (Law of Quotient).
Proof: Let logam = p and logan = q
Then, ap = m and aq = n
∴ m/n = ap/aq = ap – q
∴ p – q = loga (m/n)
⇒ loga m – loga n = loga (m/n)
(iii) The logarithm of the n-th power of a number is n times the logarithm of the number with respect to the same base i.e. logamn = n logam (Law of Exponent).
Proof: Let logam = p. Then, ap = m
∴ mn = (ap)n = apn
∴ By definition of logarithm,
pn = logamn
⇒ n logam = logamn
Note: a0 = 1 ∴ loga 1 = 0. Thus, the logarithm of 1 to any base other than zero is zero.
| Example- Prove that log2 log2 log2 log√2256 = 1. Solution- The given equation is, log2 log2 log2 log√2256 = 1 Taking L.H.S, = log2 log2 log2 log√2256 = log2 log2 log2 log(2)1/2 28 = log2 log2 log2 1/(1/2) log2 28 [∵ logbn a = 1/n logb a] = log2 log2 log2 (2 x 8) log2 2 [∵ logamn = n loga m ] = log2 log2 log2 16 (1) [∵ loga a = 1] = log2 log2 log2 16 = log2 log2 log2 24 = log2 log2 4 log2 2 = log2 log2 4 (1) = log2 log2 4 = log2 log2 22 = log2 2 log2 2 = log2 2 (1) = log2 2 = 1 |
| Example- If log (x + z) + log (x -2y + z) = 2 log (x – z) then show that 1/x + 1/z = 2/y. Solution- log (x + z) + log (x -2y + z) = 2 log (x – z) ⇒ log [(x + z) (x -2y + z)] = log (x – z)2 ⇒ x2 – 2xy + xz + xz -2zy + z2 = x2 + z2 – 2xz ⇒ 2xz – 2xy -2zy = -2xz ⇒ 2xz + 2xz = 2xy + 2zy ⇒ 2 (xz + xz) = 2 (xy + zy) ⇒ 2xz = xy + zy Divide both sides by xyz: ⇒ 2xz/xyz = xy/xyz + zy/xyz ⇒ 2/y = 1/z + 1/x |
| Example- If log (m + n) = log 2 + 1/2 log m + 1/2 log n, prove that m = n. Solution- log (m + n) = log 2 + 1/2 log m + 1/2 log n ⇒ log (m + n) = log 2 + log m1/2 + log n1/2 ⇒ log (m + n) = log [2 . m1/2 . n1/2] ⇒ m + n = 2 √(mn) ⇒ (√m)2 + (√n)2 – 2√m √n = 0 ⇒ (√m – √n)2 = 0 ⇒ √m – √n = 0 ⇒ √m = √n ⇒ m = n |
| Example- If loga b = 10 and log6a 32b = 5, find the value of a. Solution- loga b = 10 ⇒ a10 = b ……….(i) and log6a 32b = 5 ⇒ (6a)5 = 32b ⇒ 65a5 = 32 (a10) [Using (i)] ⇒ 65/32 = a5 ⇒ 65/25 = a5 ⇒ (6/2)5 = a5 ⇒ (3)5 = a5 ⇒ a = 3 |









Comments (No)