Quadratic Equations and their Solutions:
An equation of the type f(x) = 0, where f(x) is a quadratic polynomial, is called a quadratic equation. The general form of a quadratic polynomial is ax2 + bx + c, where a ≠ 0. Thus, 2x2 – 3x + 1 = 0, 4x2 + 3x = 0, 2x2 – 4 = 0 etc. are quadratic equations.
Also, equations of the type 2x2 + 3xy + y2 = 0 which may be rewritten 2(x/y)2 as 3(x/y) + 1 = 0 is a quadratic equation in (x/y).
An equation is defined as a mathematical statement showing the equality of two expressions which holds only for certain values of the letters denoting the unknown quantity involved. In the case of an equation, the equality holds for as many values of the unknown as the degree of the polynomial involved. Thus, the equation 2x – 7 = 0 has only one solution as the degree of the polynomial f(x) involved in the equation is 1. The equation 2x2 – 3x + 1 = 0 has 2 solutions as the degree of the polynomial involved is 2.
If the equality holds for more values of the unknown than the degree of the polynomial involved, then the mathematical statement would be an identity, not an equation. For example, 3x + 2 = (2x + 1) + x + 1 holds for any value of x and hence it is not an equation. Similarly, (2x -3) (3x + 2) = 6x2 – 5x – 6 is not a quadratic equation because the statement is true for any value of x, not only for two.
Solving a Quadratic Equation:
Consider the quadratic equation ax2 + bx + c = 0, a ≠ 0. Dividing both the sides by a, we get x2 + (b/a)x + (c/a) = 0.
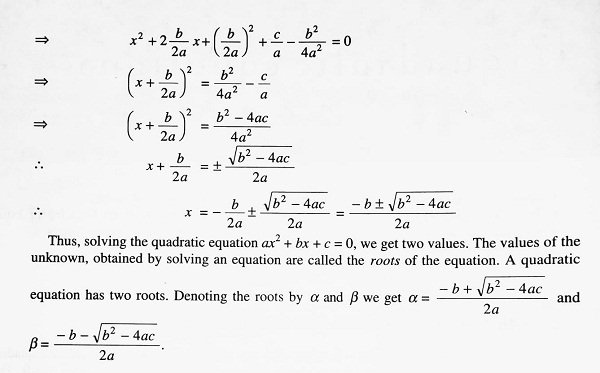








Comments (No)