Table of Contents
Dispersion of Light:
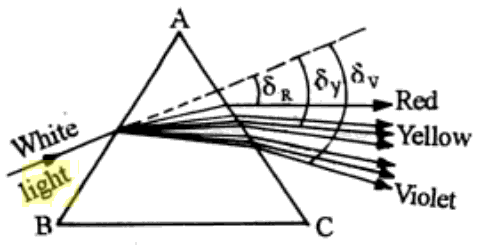
When a narrow beam of white light is allowed to pass through a prism, a beautiful pattern of colours appears on the screen, this shows that white light consists of a number of components each of which causes a particular sensation of colour. We know that light travels in waves and the components that produce different sensations of colours are infact, correspond to waves of different frequencies (or wavelengths). In a vacuum, all frequencies of light travel with the same speed c = 3 x 108 ms-1, but it is not so in a physical medium. In a medium like glass, light rays of different colours corresponding to different frequencies travel at different speeds. Since the refractive index of a medium is given by the ratio of the speed of light in a vacuum to the speed of light in that medium, it is clear that a medium has different refractive indices for different colours corresponding to different frequencies of light. Hence a prism deviates light of different colours by different amounts. The higher frequencies (smaller wavelengths) have deviated most and cause a violet sensation while lower frequencies (longer wavelengths) are deviated least and produce a red sensation in our eyes.
The phenomenon of separation of light of different frequencies (or wavelengths) into its separate components is called dispersion of light.
Cause of Dispersion of Light:
According to Cauchy’s formula, the refractive index (µ) of material depends on the wavelength (λ) as written below.
| µ = A + B/λ2 + C/λ4 +…….. ———-(i) Where A, B, C are constants. |
Also, in the case of Prism,
| δ = (µ – 1) A ———-(ii) |
| We know, λviolet < λred From (i), µviolet > µred From (ii), δviolet > δred |
Hence violet colour deviates more than red colours. The other colours suffer deviations inbetween violet and red colour.
Angular Dispersion:
Angular Dispersion is defined as the difference in the deviations produced by the two extreme colours (i.e. red and violet colour) in passing through a prism. It is denoted by θ. Mathematically,
| θ = δV – δR ———-(i) |
Expression: The deviation produced for a small angled prism is given by-
| δ = (µ – 1) A |
For red colour,
| δR = (µR – 1) A ———-(ii) |
For violet colour,
| δV = (µV – 1) A ———-(iii) |
Put (ii) and (iii) in (i), we have-
| θ = (µV – 1) A – (µR – 1) A θ = µV A – A – µR A + A θ = µV A – µR A θ = (µV – µR) A |
The unit of angular dispersion is degree.
The angular dispersion produced by the prism depends upon-
- Angle of prism.
- Nature of material of prism.
Dispersive Power of an Optical Medium:
When white light enters a transparent medium (say in form of a transparent prism) the light of different colours has deviated through different angles. Since the yellow colour lies almost near the middle, its deviation δY is used to express the mean deviation produced by a particular medium. Further (δV – δR) gives the angular dispersion caused by the medium. Both of these will be different for a different medium. The property of producing dispersion by a medium is expressed in terms of dispersive power ω which is the ratio of angular dispersion to the average deviation. These quantities are measured in the position of the prism when it is passing the mean wavelength symmetrically through it. (This is also the position of minimum deviation for this wavelength).
| Dispersive power ω = angular dispersion/average deviation = (δV – δR)/δY ———-(i) |
We may express ω in terms of refractive index (µ). For a prism of small angle A at a small angle of incidence i, the deviation produced is given by-
| δ = (µ – 1) A |
When white light passes through such a prism, the deviation of violet, yellow and red light are given by-
| δV = (µV – 1) A δY = (µY – 1) A δR = (µR – 1) A |
On substituting in equation (i) the dispersive power of the medium is found as-
| ω = [(µV – 1) A – (µR – 1) A] / (µY – 1) A = (µV – µR)/(µY – 1) ———-(ii) |
Equation (ii) itself may be taken as the definition of dispersive power. Our eye is most sensitive to the range of wavelength which lies between the F-line (sky-green, λ = 4861 Å) and C-line (red, λ = 6563 Å) of the hydrogen spectrum. The mean refractive index for this part is nearly equal to the refractive index for the D-line (Yellow, λ = 5893 Å) of sodium. Hence for dispersive power, the following relation is internationally accepted-
| ω = (µF – µC)/(µD – 1) |
The dispersive power depends only on the material of the prism and nothing else. It is the property of the medium. A prism of larger dispersive power will produce a spectrum having a wider span than a prism of lower dispersive power. The dispersive power of flint glass is more than that of crown glass.









Comments (No)